Technical Drawings

Usually, a set of technical drawings consists of a Body view, a Profile view and a Plan view.  The Plan view is sometimes called the “Seagulls’ view”.  The Plan view is divided by the longitudinal centerline into two halves that are each other’s mirror image.  For that reason the drawing can be simplified to an illustration of one side on the centerline.  It is called the half-Breadth view.  See figure 2-1.

Once you become familiar with and accustomed to the “set of plans”, it is easy to forget that they are nothing else than projections that often give a complete different, sometimes distorted picture from the reality.  To give an extremely striking example:  A straight line in a Profile and half- Breadth view can show up as just a single dot in the Body view, i.e., the center line.  Only by looking at the first two illustrations do we become aware that the dot in the Body view represents a full straight line.

Another example is that a circle can show up as a straight line in two projection planes.  Only the third projection will give us the real picture.

Plans of a Double-Ender

Figure 2-1 shows the three projection drawings of a 16-ft. Double-Ender with constant flare.

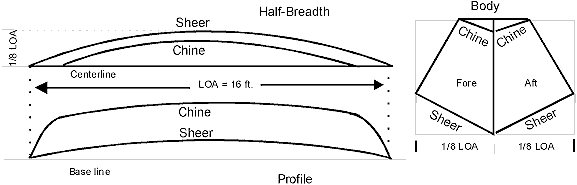
Fig. 2 - 1   Set of plans of a Double-Ender

In de Half-Breadth and Profile view the sheer lines show up as parts of elliptical curves.  In the Body view that sheer line is a straight line!

Also, the sheer line in the Body view is the hypotenuse of a right triangle.  That right triangle conforms to the other right triangle above it on the side.  The hypotenuse of this second right triangle is the side panel of the hull.  The angle between the long leg and the hypotenuse of that right triangle represents the constant flare angle of the side panel.

In the right triangle of which the sheer line is the hypotenuse, the horizontal (long) leg represents the width of the half-Breadth at Beam in the half-Breadth view.  This width is 1/8 LOA = 24".

The vertical (short) leg of this triangle is the Profile height at Beam in the Profile view.  IF that Profile height is 10", the hypotenuse/sheer line is 26" according to the theorem of Pythagoras (a2  = b2  + c2).

The late John Gardner states on page 43 in The Dory Book that the sheer line of the Double-Ender is a “natural curve”.  By definition that means “part of a circle (arc).”

A chord is a straight line on which a circle arc stand.  The half-Breadth view shows us clearly that chord.  It is the centerline of the drawing.  The length of the chord = the length of the centerline = the Overall Length:  LOA =16 ft. = 192"

To draw the real sheer line has now become the grade-8, secondary school, mathematical problem how to draw a circle arc on a chord when you know the length of the chord, and the height of the arc above the chord.

Rephrased, the real question is: Calculate the radius of a circle arc standing on a chord.  The chord is 192", the height of the arc is 26".

I obtained my high school certificate in 1939.  Since that time, all that I had ever used from all the mathematics that I had crammed in high school was Ohm’s Law, which is expressed by the formula V = I x R.  I had needed the formula to figure out the rating to replace a blown-out Xmas-tree light in a series string.  My knowledge of mathematics had stifled, and rusted over in a far away corner of the brain box.  I searched some old school books that my by-this-time-married children had left behind.  But having learned mathematics in Holland, I had a hard time with the expressions of the English language equivalents in 1990, 50 years later.  I experienced one of the very few moments that I felt lost.

An accidental fall into a seven-feet deep hole landed me on a concrete basement floor and subsequently in the hospital with a broken Ischium bone.  In the other bed was a cabinetmaker with a locked shoulder.

He read a woodworker’s magazine.  I was reading WoodenBoats.  Hospital days are very long, especially if you are not real sick.  The next day we switched magazines.  On page 4 was the solution of my problem.  All you need is to apply the Pythagorean equation.  See figure 2-2.  The applicable numbers are already put in

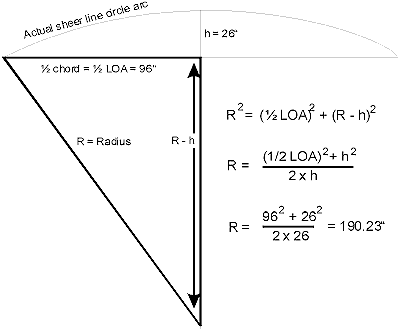
Fig. 2 - 2   Radius of a circle arc segment on a chord

THE KEY

Do I hear the question:  Now that you can calculate the radius of the sheer line circle arc, SO WHAT? … 

In the month that I was forced to sit out the healing of my broken bone, I discovered that once I knew the radius of the sheer line, it is easy to figure out:

1.   The height of the arc at each station.  That determines the exact location of the station lines in the Body view.  They can be drawn in into figure 2-1.  See figure 2-3.

2.   Once you do that, all the values of the Profile heights and half-Breadth at the stations are at your fingertips.  You can compile the offset table of the hull lines

3.   The exact real bevel angles of the cross frames and the transom.

4.   The width at each station, and the total length of the expanded side panels

5.   The length, the shape, the rake, and the crosscut angle of the (bow) stem).

6.   The measurement and the shape of the bottom.

What is more important:  It was no longer necessary to calculate any of these figures.  A full-sized Body view drawing reveals all this information.  Take off the measurement with a dividers compass.  Transfer them to the building material directly.  A method that is far more accurate and certainly faster than measuring with a ruler and loft the hull from the offset table.  Profile and half-Breadth drawings have become redundant.  Offset tables, often a primary cause of troubles, are no longer needed.

A successful project starts with an accurate technical drawing.

My technical drawings are reduced to a circle arc on a chord, and a right triangle.

You can’t go far wrong with that, or improve the degree of accuracy!  Even if the drawings are scaled down to the easy to handle size of ¼, a high degree of accuracy can be achieved.  On top of that, IF you know how to use the Staedtler-Mars scale (ruler) #987 18-34 and make the drawing on one-inch-grid graph paper, you can use the same figures from the full-sized illustration.  No conversion calculations, not the slightest chance of making mistakes.  Practically, a foolproof method.

My “SET OF PLANS” for The Double-Ender

Figure 2-3A and B are just that.  In figure 2-3B, the locations of the station lines are plotted onto the hypotenuse.  Since the vertical middle line divides the drawing into two mirror-image-equal halves only one half is drawn, just as the half-Breadth drawing replaces a full Plan view illustration.

In figure 2-3B the long, 24", horizontal leg of the right triangle is the half-Breadth at Beam.  The vertical, 10", short leg is the Profile height at Beam.

The 26" hypotenuse represents hn = hBeam = h8.

For clarity, only the locations of the even-numbered station lines are plotted on the hypotenuse.   Besides that, more frames are seldom necessary.

If both drawing are made on one-inch-grid graph paper, the numbers of the offset tables can be “read-off” from the drawing.  As mentioned before, it is a lot quicker and more accurate to transfer the dimensions to the material with a dividers compass.  Good bye and good riddance offset tables!

If any of the readers can shows me a better, faster, and more accurate way, IMHO, I think, we all like to hear about that.

The drawings also make a Profile and a half-Breadth view redundant, be it with one definite and one possible exception.

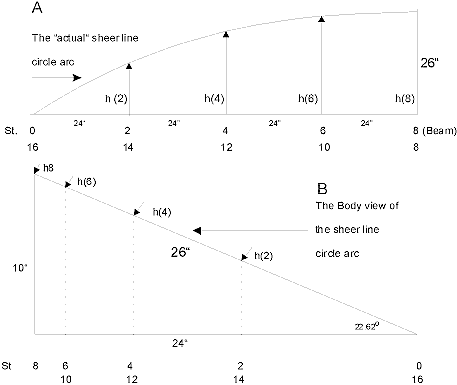
Fig. 2 - 3.   Actual sheer line circle arc (A)
Body view of the sheer line arc (B)

Note: The drawing A and B are made to scale.  However, for clarity, the scale of drawing B is 4 times the scale of drawing A

The Exceptions:  Bow stem and Transom

The real shape of the bow stem can be seen only in the Profile view.  Also, if the hull has a raked transom, its real length and rake angle only show up in the Profile view.

Before this is explored, you have to get acquainted with a couple of other short cuts that facilitate building a hard-chined hull.  That will be shown and discussed later.

Cross Frame Bevel Angles

To obtain the maximum “bearing” surface for a good connection to the side panels, the side edges of the cross frames and the frame linings are beveled.  See figure 2-4.  A skilled carpenter has no difficulty with that.  If it does not fit perfectly, a couple of strokes with a plane, et voila´.

Plywood edges and narrow lining battens are difficult to plane.  Beginning amateurs usually don’t have good carpenter’s skills.  I should know, because I don’t have them still.

But the skill is not necessary.  The bevels are easily cut with a table saw.

Never take the bevels from the half- Breadth view.  They are always wrong.

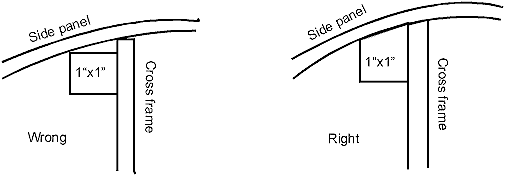
Fig. 2 - 4   Side panel “bearing” on cross frame

A bevel angle of a cross frame is equal to the center point angle between the radius of that particular station and the radius at Beam.  See figure 2-5.  

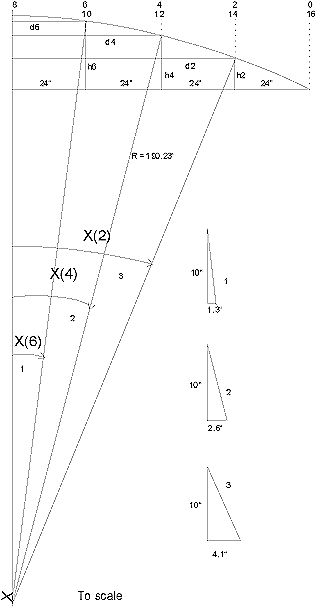
Fig 2 - 5   The cross frame bevel angles are equal to the center point angles

The sine of the center point angle X4 is Sin angle X4 = d4/R = 48/190.23 = 0.252326.  The angle X4 = 14.615º.  In the same way, Sin angle X2 = d2/R = 72/190.23 = 0.3785.  The angle X2 = 22.24º, and Sin angle X6 = d6/R = 24/190.23 = 0.126.  The angle X6 = 7.25º

The pleasant thing is that you don’t need to do any calculation!  Transfer the size of each center point angle of the actual circle arc drawing to a triangular piece of scrap plywood as shown in the drawing.  Place that right-triangular template with its short leg on the table.  Tilt the saw against the hypotenuse.  The bevel cuts will be “dead on”.  Cutting the templates goes faster than making the calculation.

Mark the templates clearly, because they are needed for #10 frame (= #6), #12 frame (= #4), and  #14 frame (= #2).  That way, the bevel angles of the corresponding frames are perfectly equal.

Sheers and Chines, Barend.